![]()
 Home
Home
![]()
Try this at home!
What crushed this can?
S.M.U. Physics
The can implodes because the atmospheric pressure is greater than the pressure inside the can.
When the can was submerged in the water it caused the water vapor inside the can to rapidly cool. When a gas is cooled, its pressure goes down. This drop in pressure causes the pressure inside the can to be lower than the atmospheric pressure.
Note: Use less than 1 cm of water in this experiment (about 1/2 inch)
Elastic P.E. - work stored in a deformed spring
a) General Equation
PEs = Fx
F = Average Force needed to deform spring
x = distance deformed (stretch or compress)

Ideal Spring
St. Mary's H.S.
b) Hooke's Law - force needed to deform an ideal spring, a given amount is directly proportional to its displacement (deformation)

Graphic from Learning-Connections Clipart
![]()
Robert Hooke
'Newton's bitter rival'
![]()
Ex) Ideal Spring
|
F
(Force to Deform) (N) |
X
(distance deformed) (m) |
|
2.0 |
.30 |
| 4.0 | .60 |
|
6.0 |
.90 |
|
8.0 |
1.2 |
![]()
University of Colorado - PhET
![]()
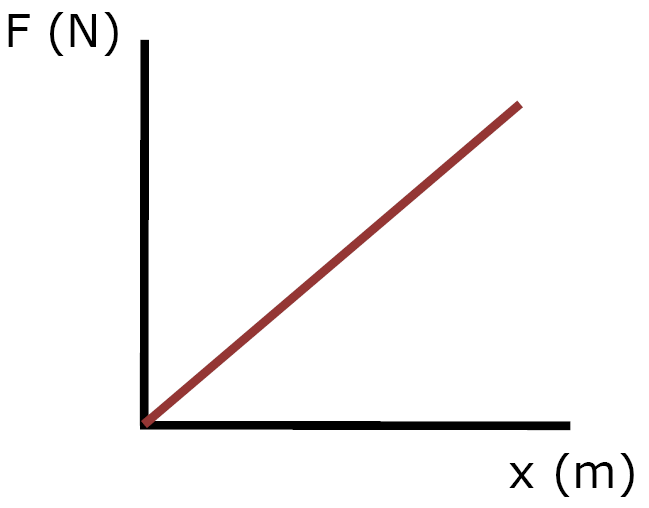
slope = F/x
x = stretch
c) Spring Constant (k), Ideal Spring
- quantifies stiffness of a spring
| K | = | F | (N) |
| X | (m) |
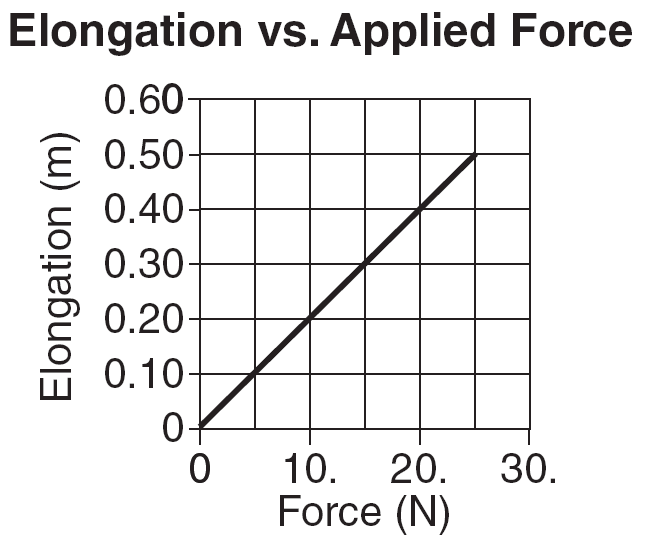
What is the spring constant of the spring that produced the data? above?
| F
(Force to Deform) (N) |
X
(distance deformed) (m) |
| 2.0 | .30 |
| 4.0 | .60 |
| 6.0 | .90 |
| 8.0 | 1.2 |
| k | = | 2.0 N |
| .30 m |
k = 6.7 N/m
Which is the stiffer spring?
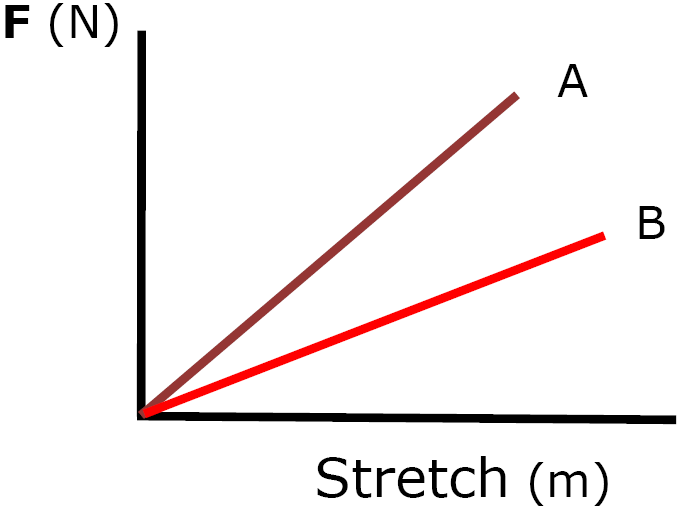
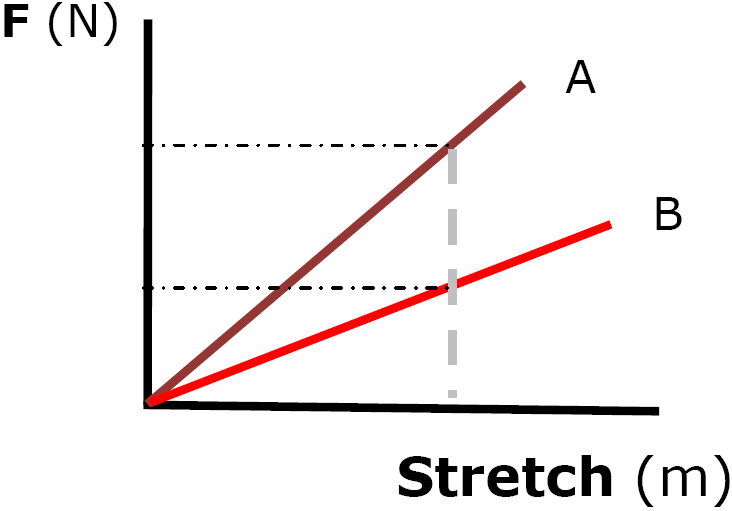
Spring A is the stiffer spring.
More
force is required
for the same stretch

Ex) Plots and K
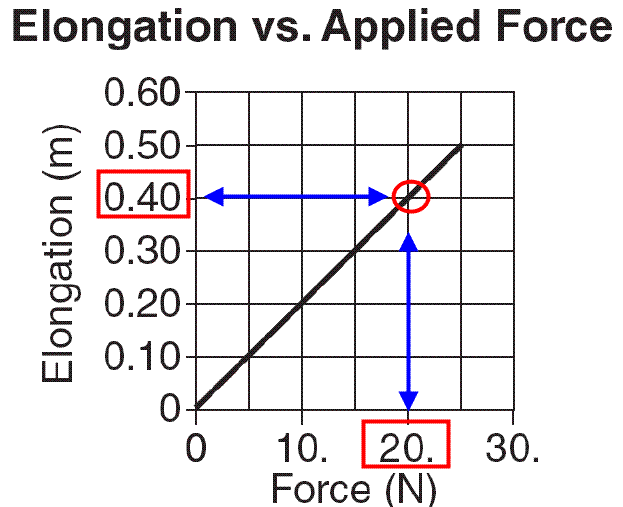
Pick any point on the line
| K | = | F | (N) |
| X | (m) |
| K | = | 20. N |
| .40 m |
K = 50. N/m
31) A spring with k = 53 N/m hangs
vertically on a ruler. The end of the spring is next to the 15 cm mark
on the ruler.
If a 2.5 kg mass is now attached to the end of the spring, where will
the end of the spring line up with the ruler marks?
k = F/x
53 N/m = 2.5kg[9.8m/s2]/x
x = .46 m + .15 m
ruler reading
= .61 m
d) Elastic P.E. = area of triangle under F vs x graph
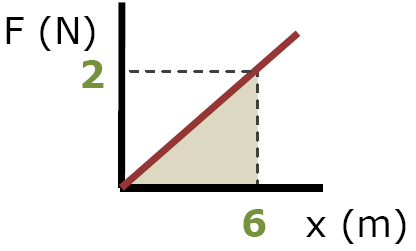
PEs = (½)basexheight
PEs = 6 Joules
![]()
![]()
Try this at home!
©Tony Mangiacapre.,
- All Rights Reserved [Home]
Established 1995
Use any
material on this site (w/ attribution)